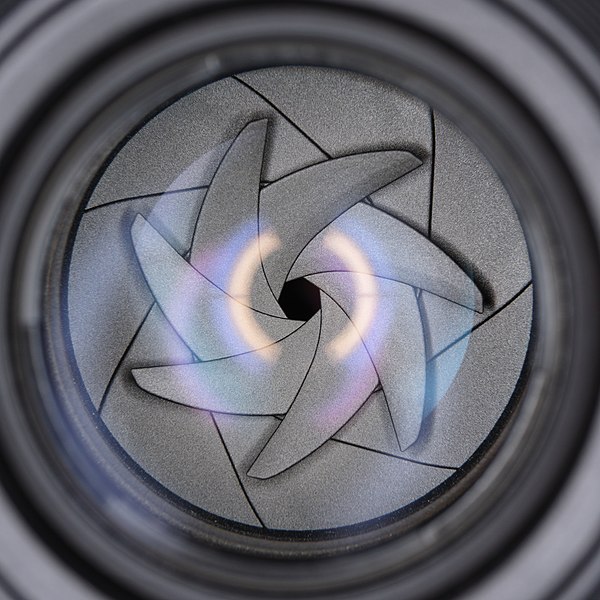
In optics, the aperture of an optical system is a hole or an opening that primarily limits light propagated through the system. More specifically, the entrance pupil as the front side image of the aperture and focal length of an optical system determine the cone angle of a bundle of rays that comes to a focus in the image plane.
Different apertures of a lens
In biology, the pupil (appearing as a black hole) of the eye is its aperture and the iris is its diaphragm. In humans, the pupil can constrict to as small as 2 mm (f/8.3) and dilate to larger than 8 mm (f/2.1) in some individuals.
A camera aperture
Alvin Clark polishes the big Yerkes Observatory Great Refractor objective lens, with 40 inches 102 cm across, in 1896.
Optics is the branch of physics that studies the behaviour and properties of light, including its interactions with matter and the construction of instruments that use or detect it. Optics usually describes the behaviour of visible, ultraviolet, and infrared light. Light is a type of electromagnetic radiation, and other forms of electromagnetic radiation such as X-rays, microwaves, and radio waves exhibit similar properties.
A researcher working on an optical system
The Nimrud lens
Reproduction of a page of Ibn Sahl's manuscript showing his knowledge of the law of refraction
The first treatise about optics by Johannes Kepler, Ad Vitellionem paralipomena quibus astronomiae pars optica traditur (1604), generally recognized as the foundation of modern optics.