Douglas Engelbart
Videos
Page
Douglas Carl Engelbart was an American engineer and inventor, and an early computer and Internet pioneer. He is best known for his work on founding the field of human–computer interaction, particularly while at his Augmentation Research Center Lab in SRI International, which resulted in creation of the computer mouse, and the development of hypertext, networked computers, and precursors to graphical user interfaces. These were demonstrated at The Mother of All Demos in 1968. Engelbart's law, the observation that the intrinsic rate of human performance is exponential, is named after him.
Engelbart in 2008
Engelbart's prototype of a computer mouse, as designed by Bill English from Engelbart's sketches
Two Apple Macintosh Plus mice, 1986
SRI International
Videos
Page
SRI International (SRI) is an American nonprofit scientific research institute and organization headquartered in Menlo Park, California. The trustees of Stanford University established SRI in 1946 as a center of innovation to support economic development in the region.
Entrance to SRI International headquarters in Menlo Park
SRI participant Paul Magill discussing the smog on Black Friday in Los Angeles at the first National Air Pollution Symposium in 1949
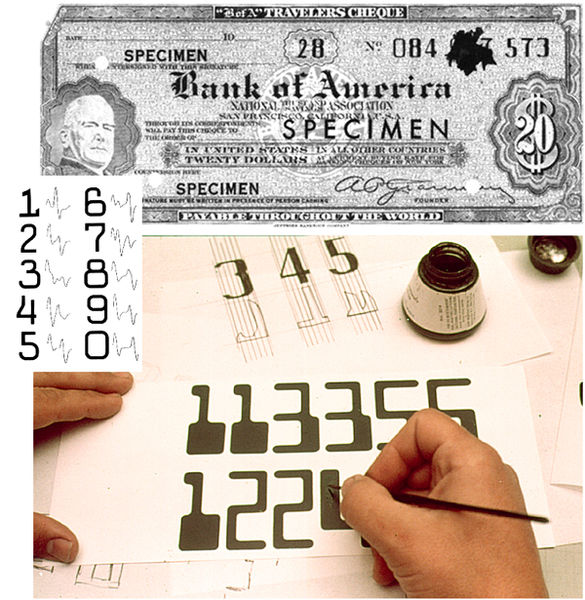
The ERMA system, which uses magnetic ink character recognition to process checks, was one of SRI's earliest developments.
The first prototype of a computer mouse, as designed by Bill English