In mathematics, a Misiurewicz point is a parameter value in the Mandelbrot set and also in real quadratic maps of the interval for which the critical point is strictly pre-periodic. By analogy, the term Misiurewicz point is also used for parameters in a multibrot set where the unique critical point is strictly pre-periodic. This term makes less sense for maps in greater generality that have more than one free critical point because some critical points might be periodic and others not. These points are named after the Polish-American mathematician Michał Misiurewicz, who was the first to study them.
Image: Orbit of critical point z=0, under f( 2),
Image: MIS1
Image: Misi 13limb
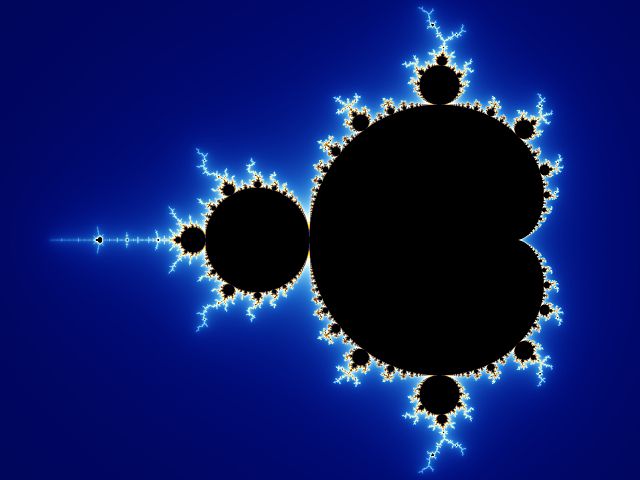
The Mandelbrot set is a two-dimensional set with a relatively simple definition that exhibits great complexity, especially as it is magnified. It is popular for its aesthetic appeal and fractal structures. The set is defined in the complex plane as the complex numbers for which the function does not diverge to infinity when iterated starting at , i.e., for which the sequence , , etc., remains bounded in absolute value.
The Mandelbrot set within a continuously colored environment
Start. Mandelbrot set with continuously colored environment.
Gap between the "head" and the "body", also called the "seahorse valley"
Double-spirals on the left, "seahorses" on the right